Plots and Results, Winter conferences 2005
(including all experimental results presented at
Moriond 2005 and CKM 2005, San Diego)

Newest and more plots and results can be found in this seminar: ppt file (SLAC, May 2005)

Menu:

Numerical results:

A brief document that provides the collection of up-to-date inputs to the global CKM analysis and the numerical results found. The results include: Wolfenstein parameters, UT angles, (combinations of) CKM elements, theory parameters and rare branching fractions. Detailed background information on the methodology and the treatment of experimental and theoretical uncertainties is provided in hep-ph/0406184.
ps

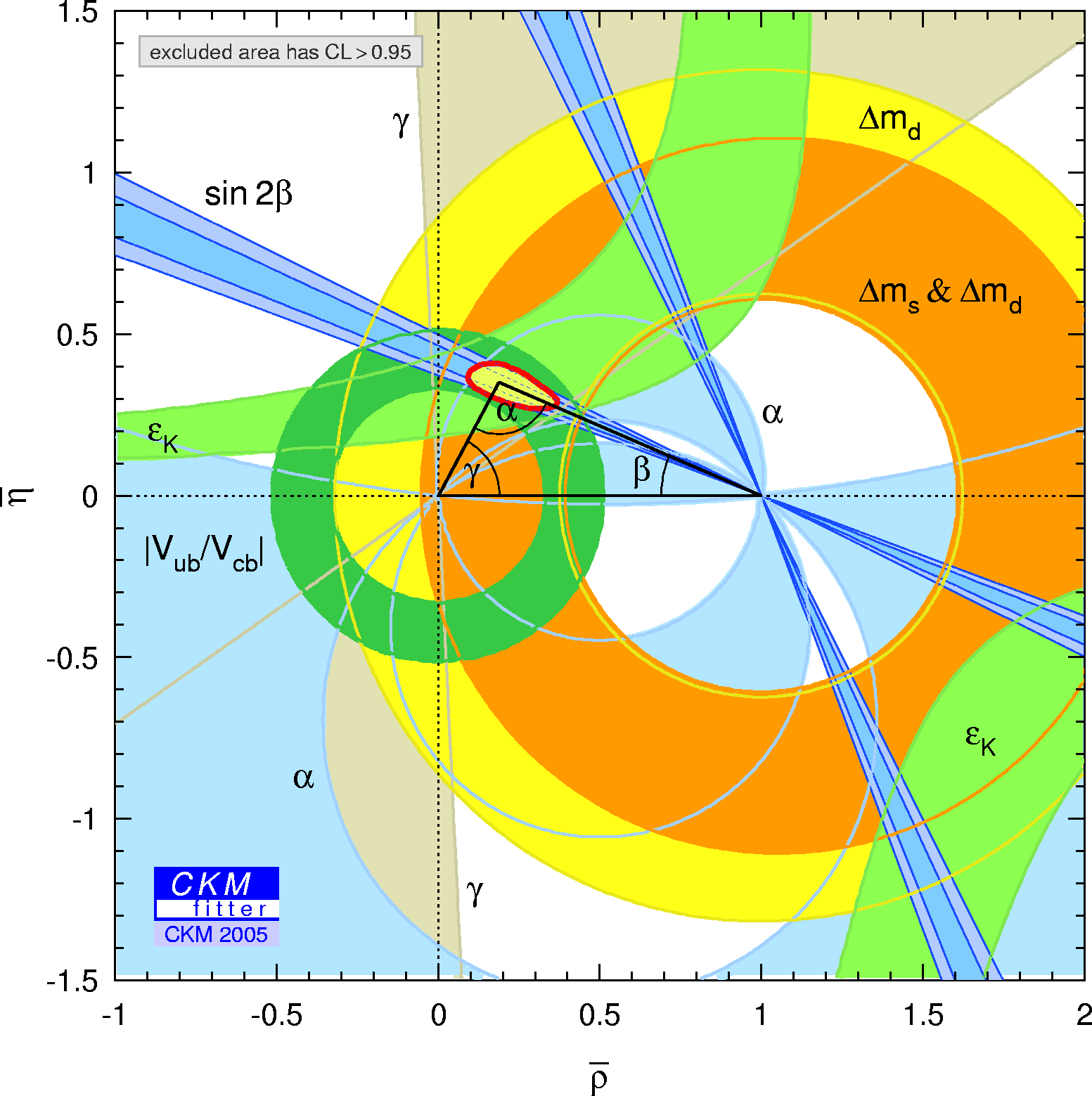
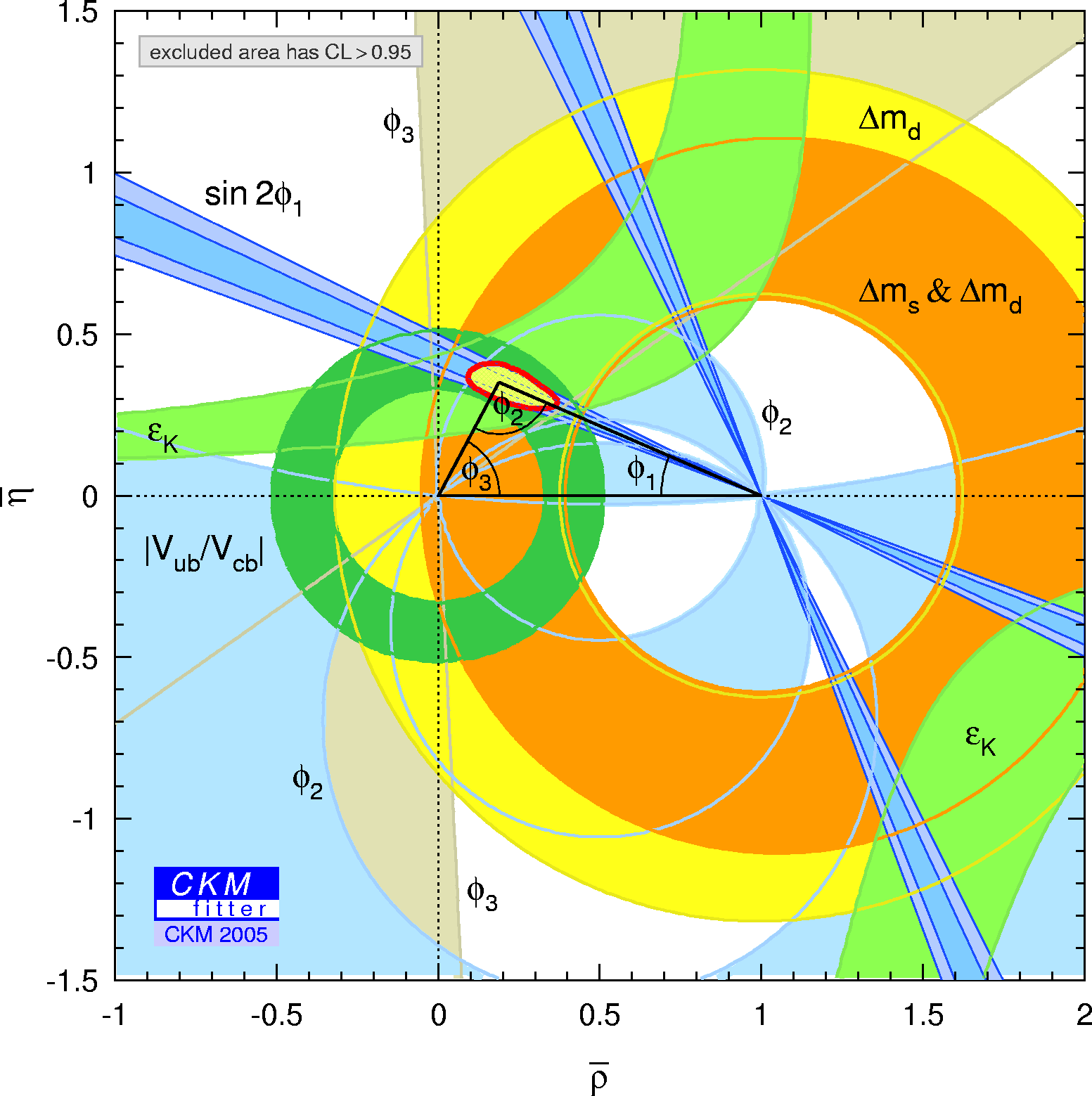
The global CKM fit in the large (ρ-bar,η-bar) plane:

α,β,γ convention: φ1,φ2,φ3 convention:
Constraints in the (ρ-bar,η-bar) plane including the most recent α/Φ2- and γ/Φ3-related inputs in the global CKM fit.
eps gif
eps gif
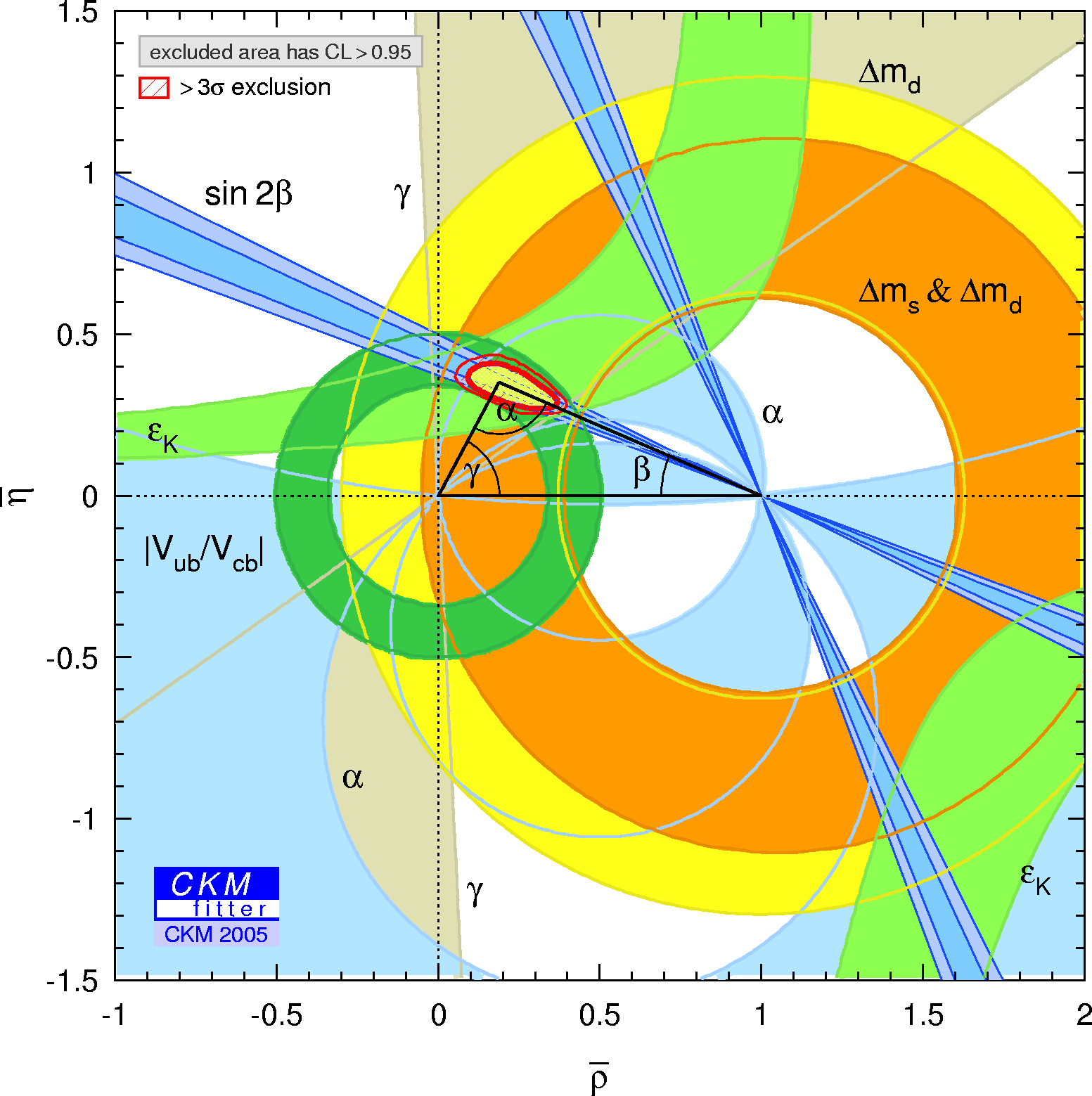
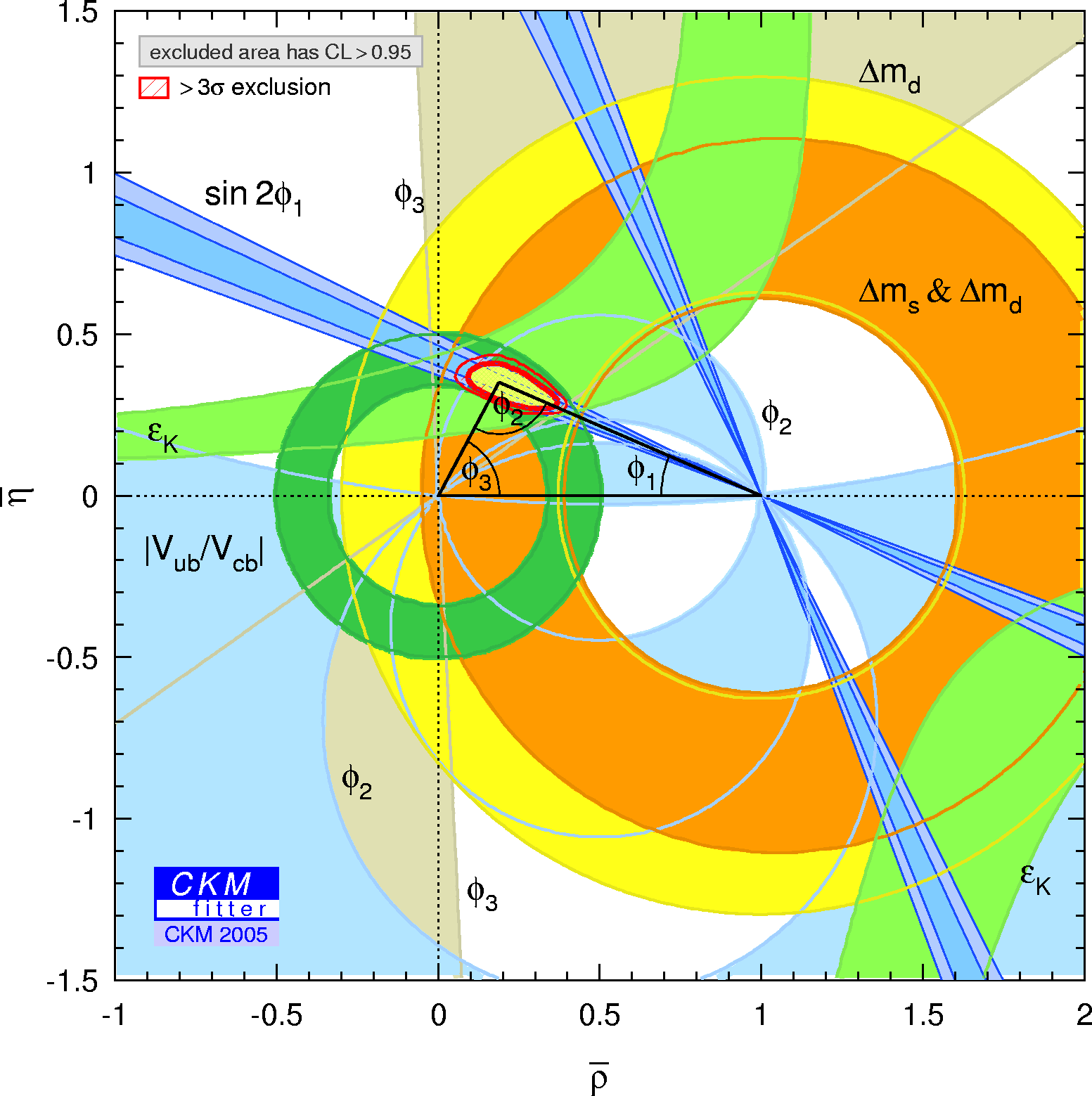
Same as above, and also showing the 3σ contours of the global CKM fit.
eps gif
eps gif
Constraints in the (ρ-bar,η-bar) plane not including the angle measurements in the global fit.
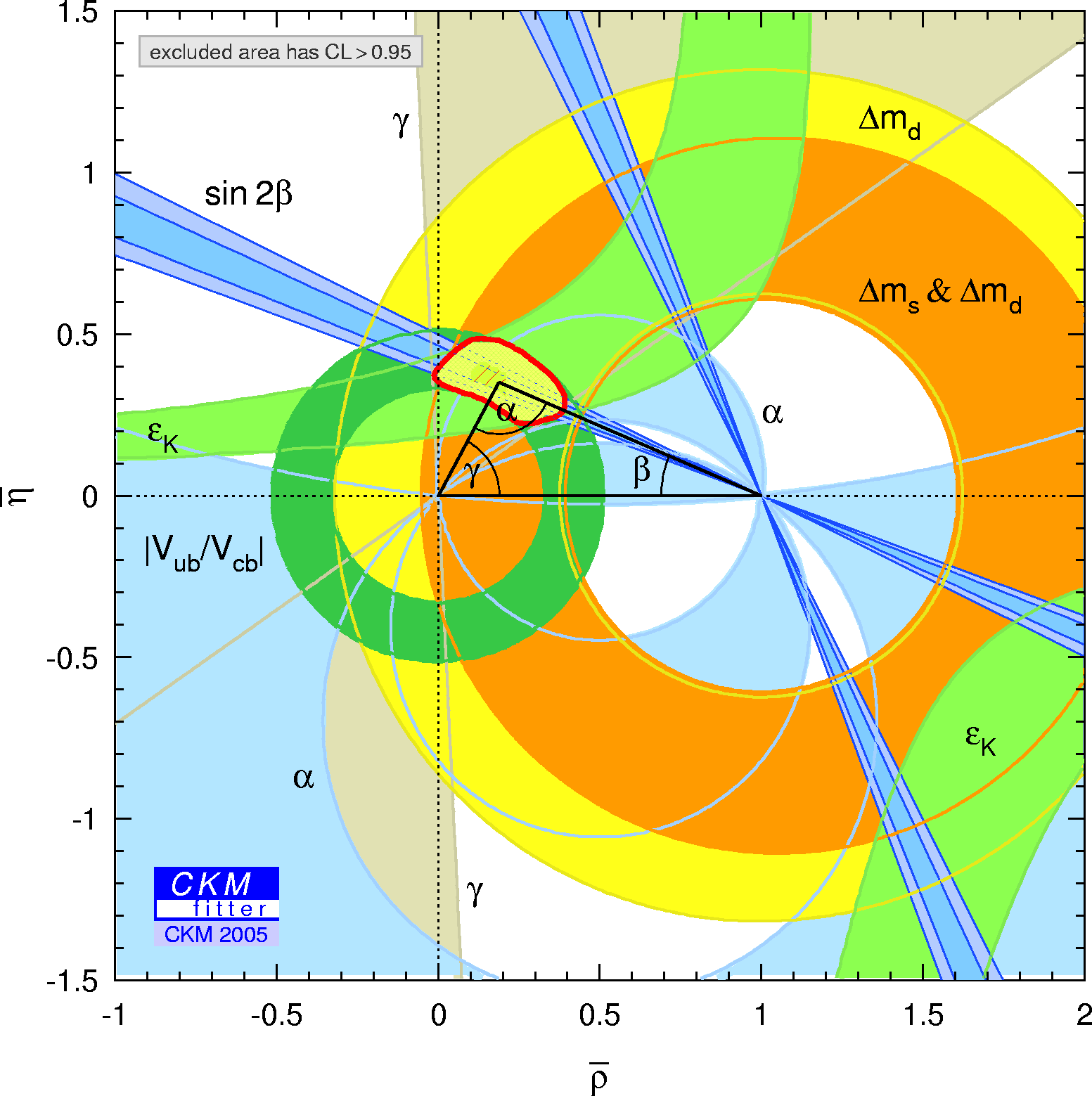eps gif
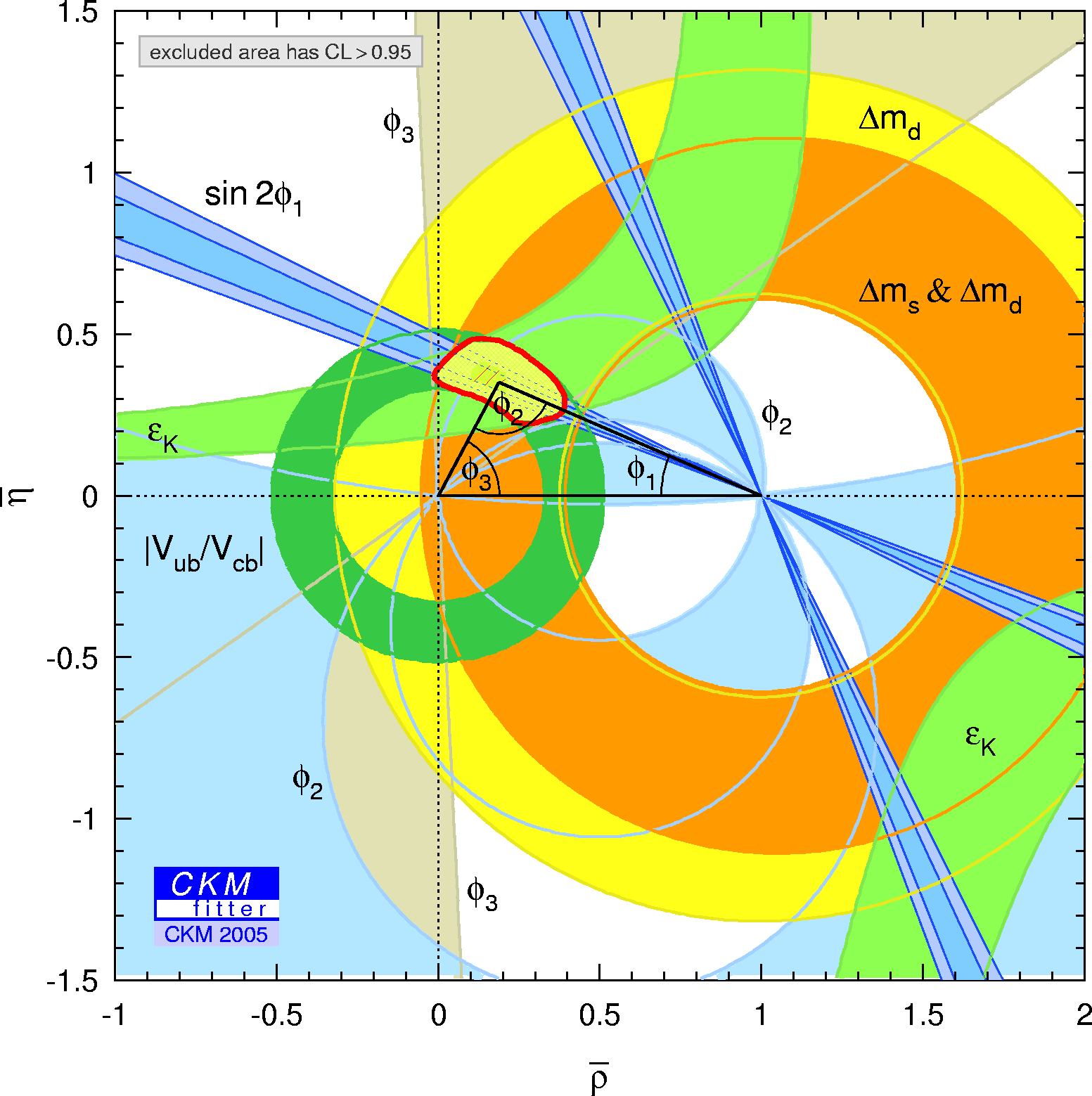eps gif
Constraints in the (ρ-bar,η-bar) plane including only the angle measurements in the global fit.
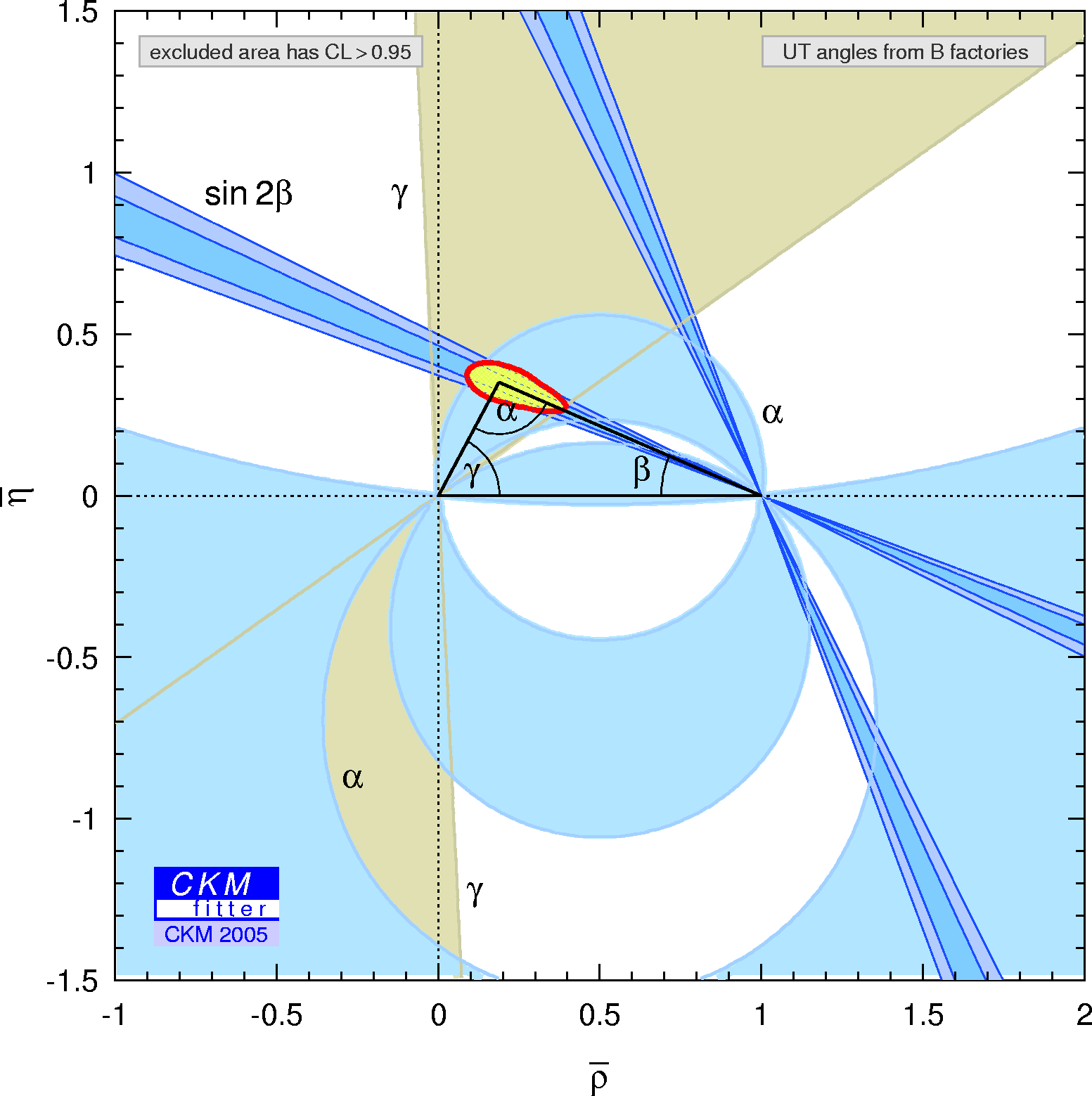eps gif
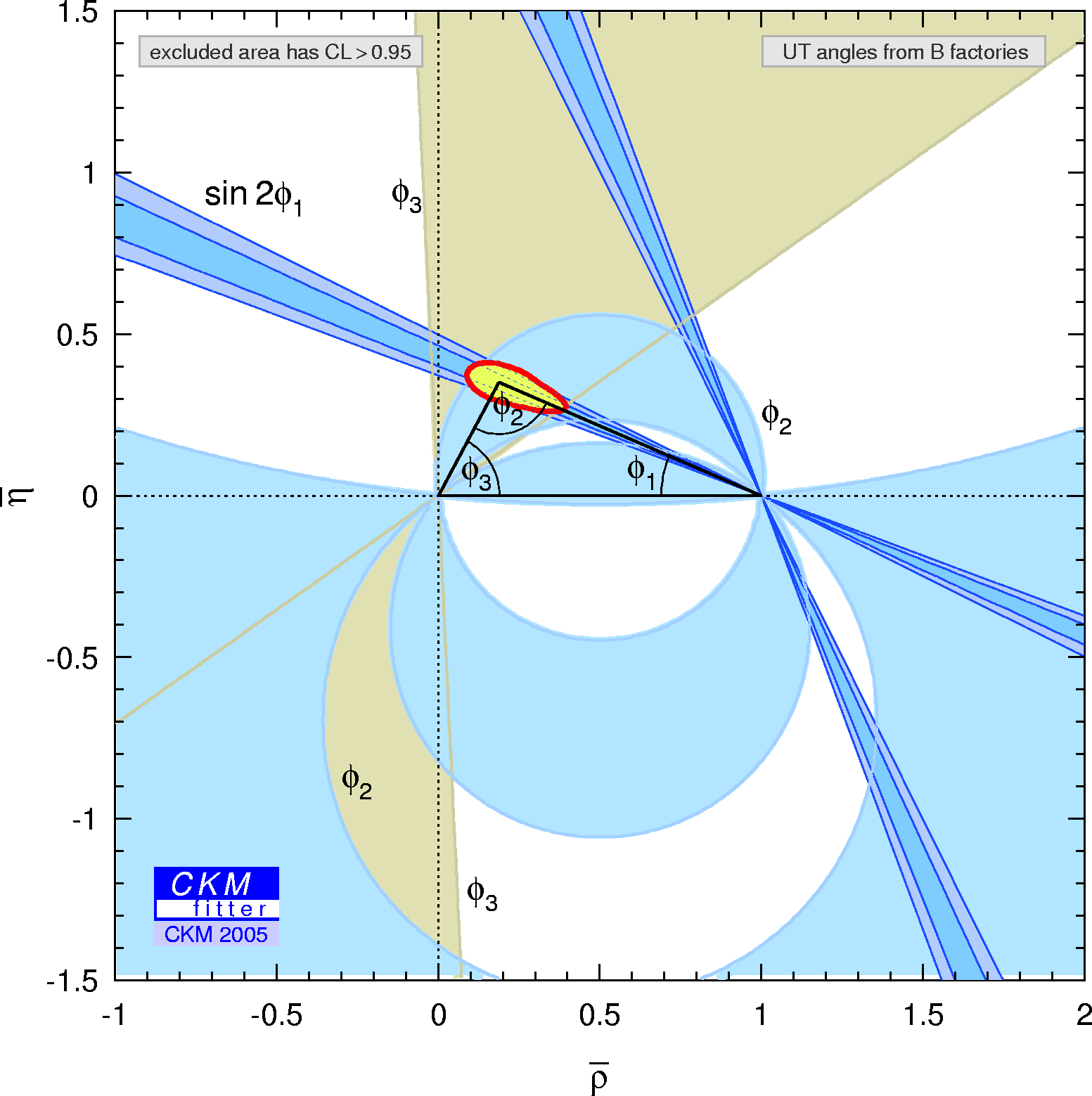eps gif
Constraints in the (ρ-bar,η-bar) plane including only the angle measurements in the global fit, and also showing the 3σ contours of the fit.
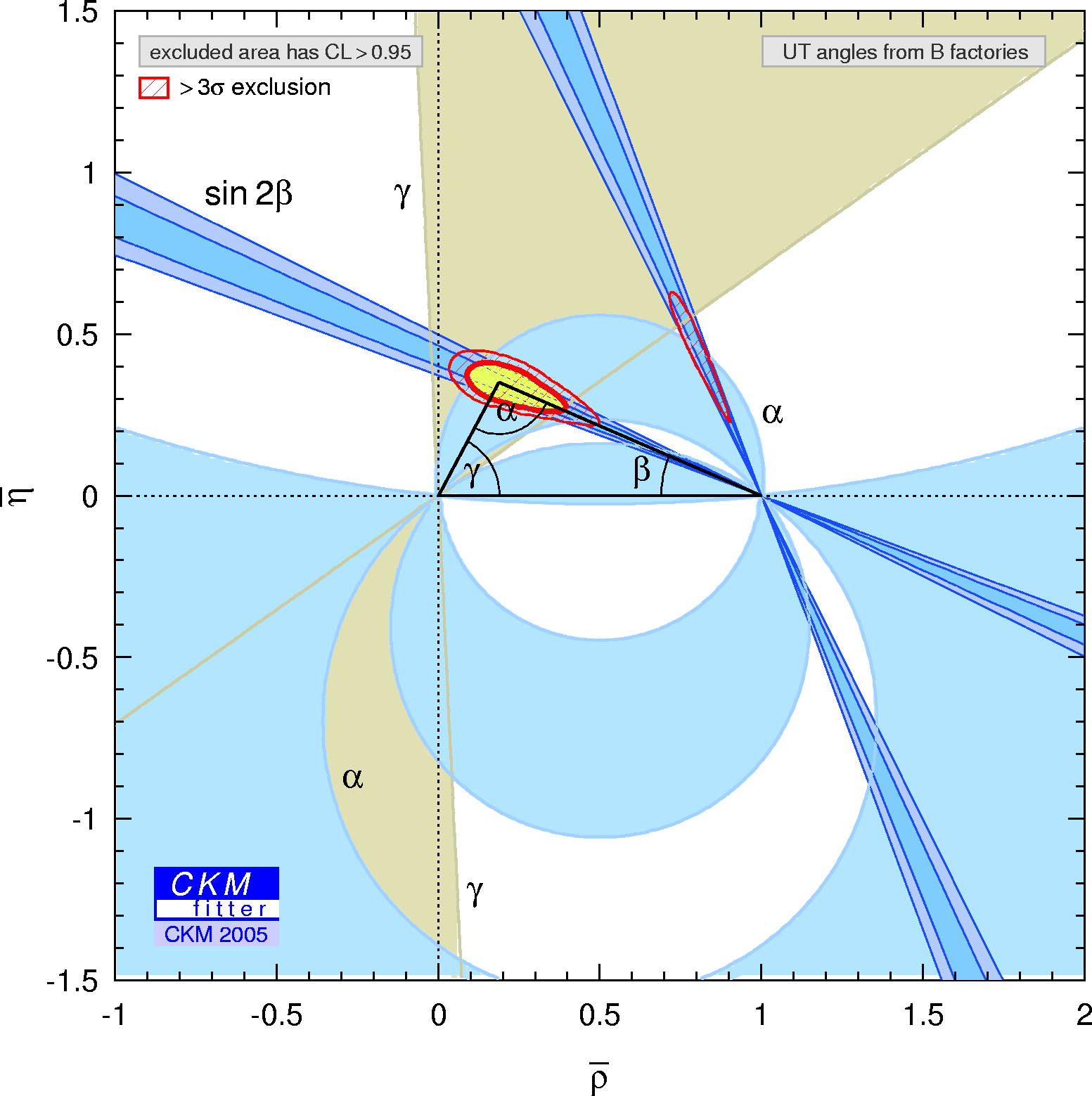eps gif
eps gif

The global CKM fit in the small (ρ-bar,η-bar) plane (zoom):

α,β,γ convention: φ1,φ2,φ3 convention:
Zoomed constraints in the (ρ-bar,η-bar) plane including the most recent α/Φ2- and γ/Φ3-related inputs in the global CKM fit.
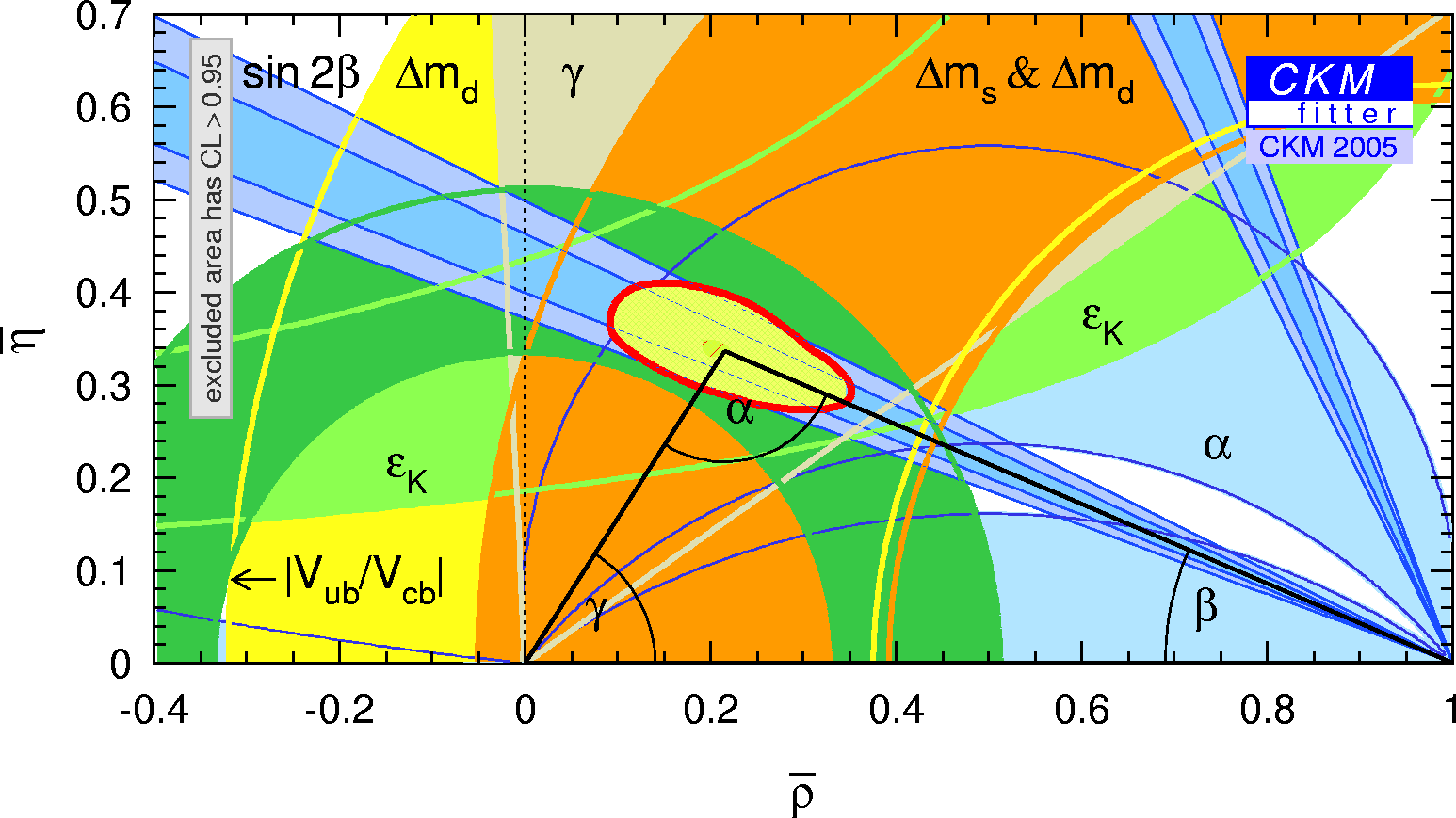eps gif
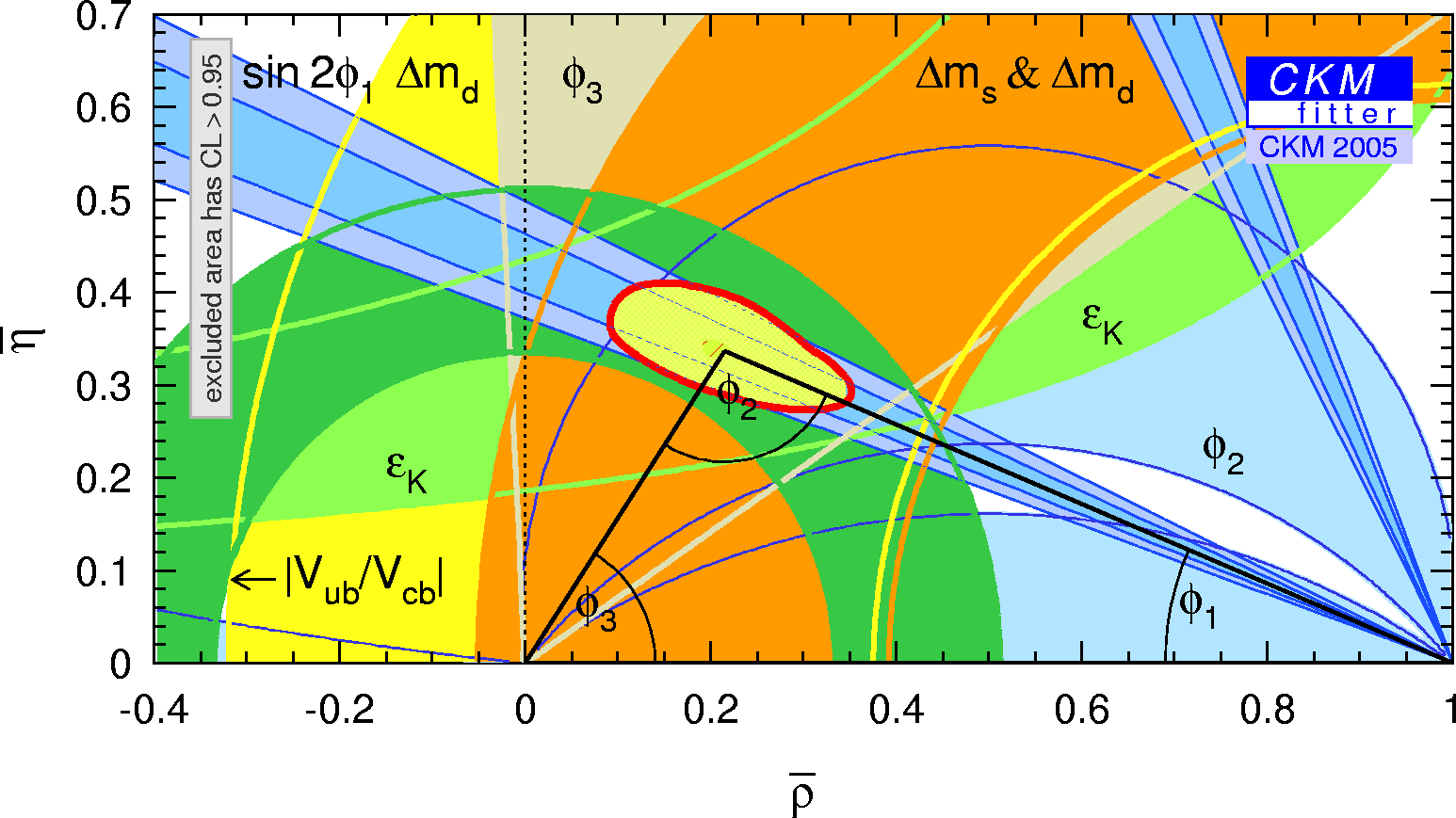eps gif
Zoomed constraints in the (ρ-bar,η-bar) plane not including the angle measurements in the global fit.
eps gif
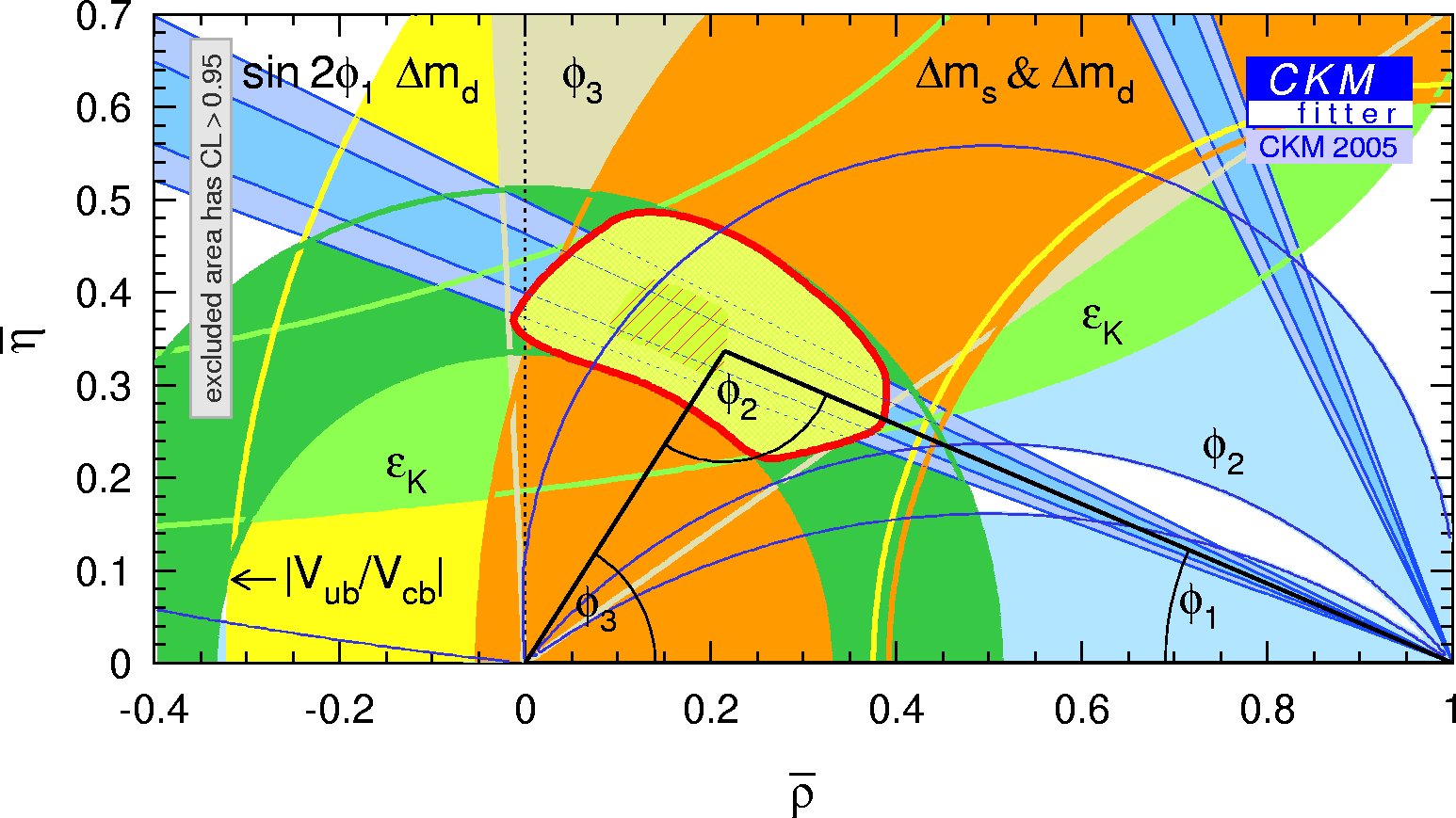eps gif

Constraints on the angle α/Φ2 from charmless B decays:

Constraint on α/Φ2 from B→ππ separatley for BABAR and Belle, and both combined. The light shaded region indicates the combined constraint when not using C(π0π0) in the isospin analysis.
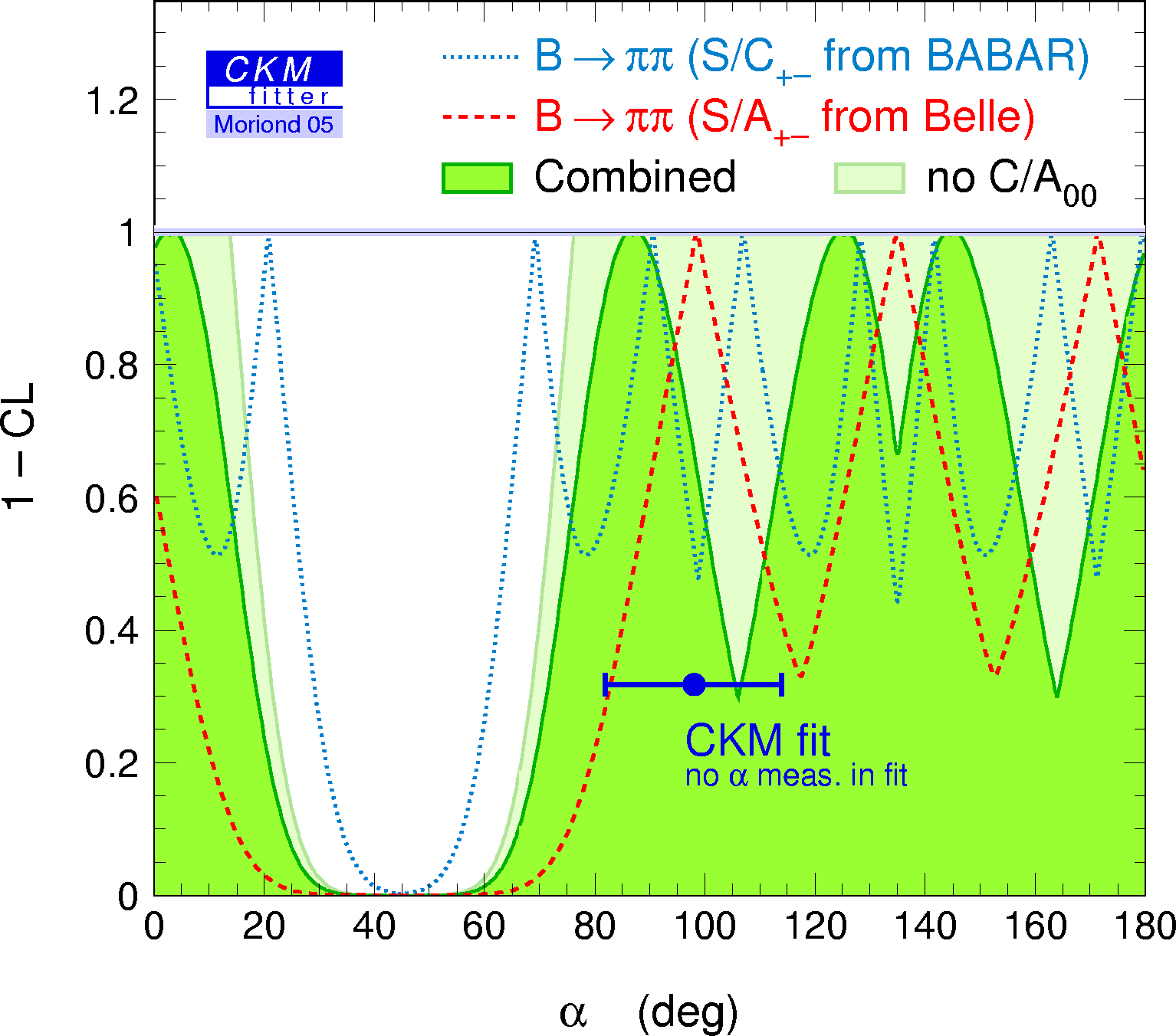eps gif
Constraints on α/Φ2 from B→ππ, ρπ, ρρ, compared to the prediction from the CKM fit (not including these measurements).
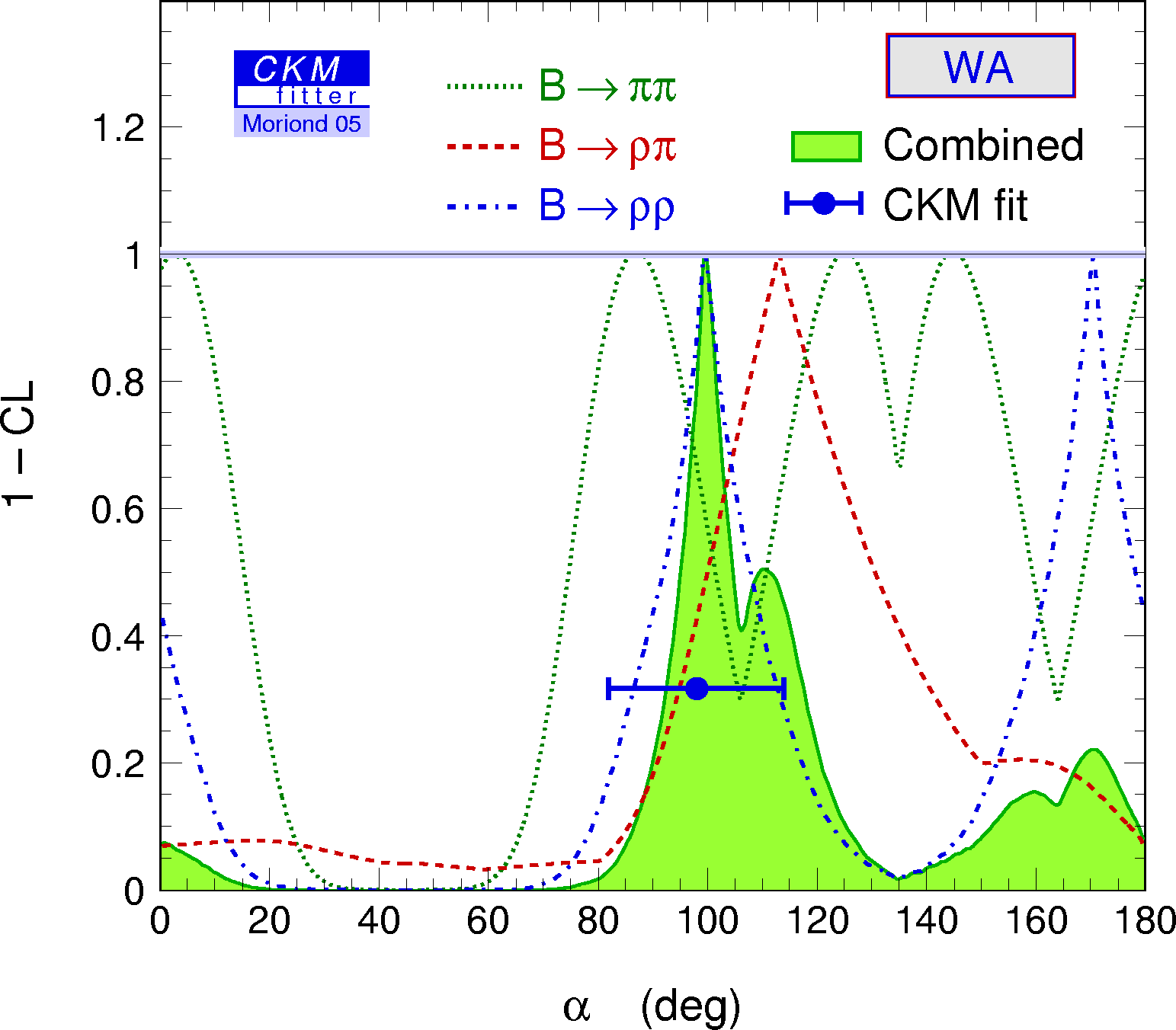eps gif
Constraint in the (ρ-bar,η-bar) plane from B→ππ, ρπ, ρρ, compared with the global CKM fit (not including these α-related measurements).
eps gif

Constraints on the angle γ/Φ3 from B decays to charm:

Constraints on γ/Phi;3 from D(*)K decays (GLW+ADS and Dalitz analyses) compared to the prediction from the global CKM fit (not including these measurements).
γ[GLW+ADS+GGSZ] = 63 +15 / –13°
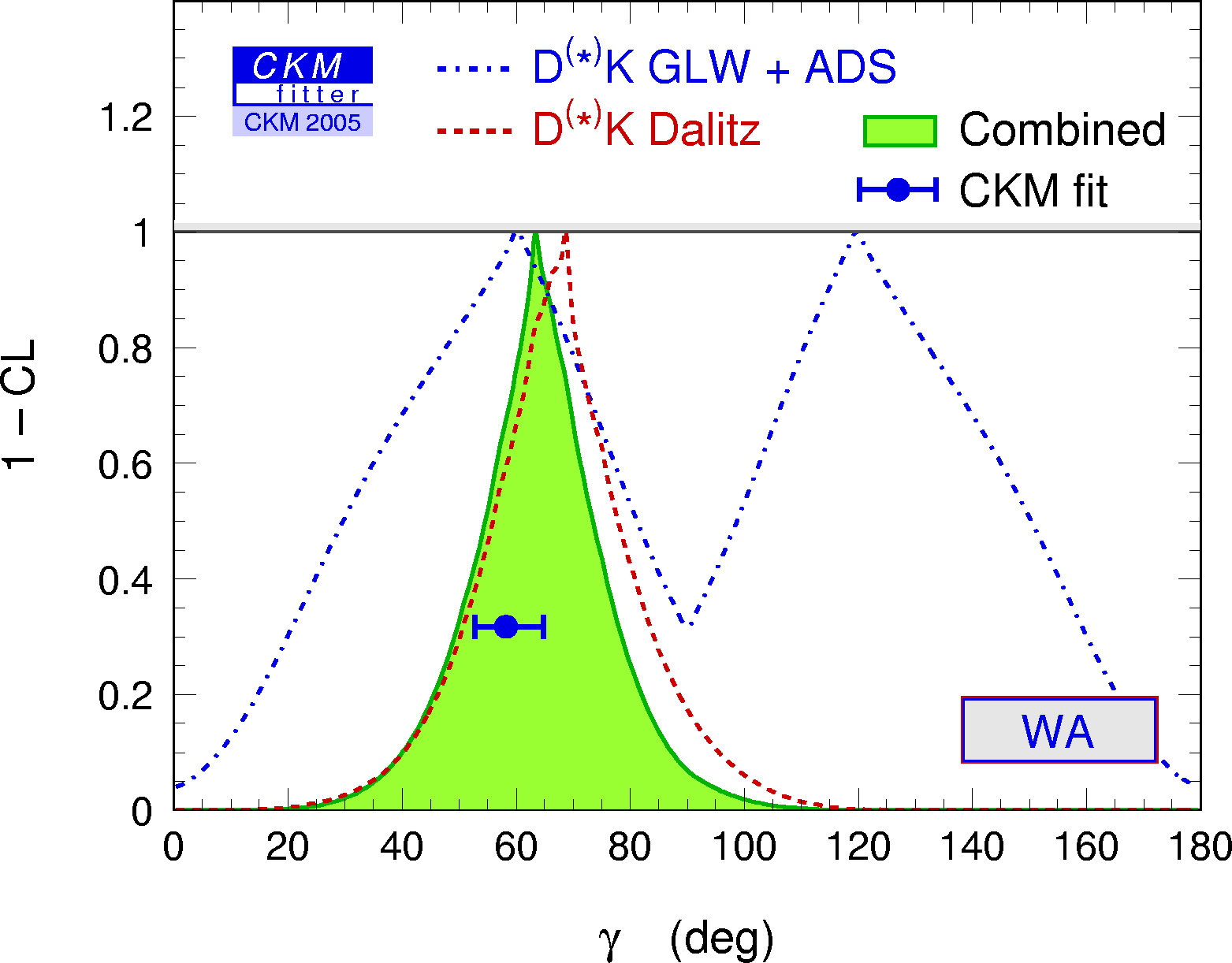eps gif

Constraint from limit on the B+→τ+ ν branching fraction (recent result from BABAR)

Constraint in the (ρ-bar,η-bar) plane from the simultaneous use of the limit on the B+→τ+ν branching fraction and Δmd.
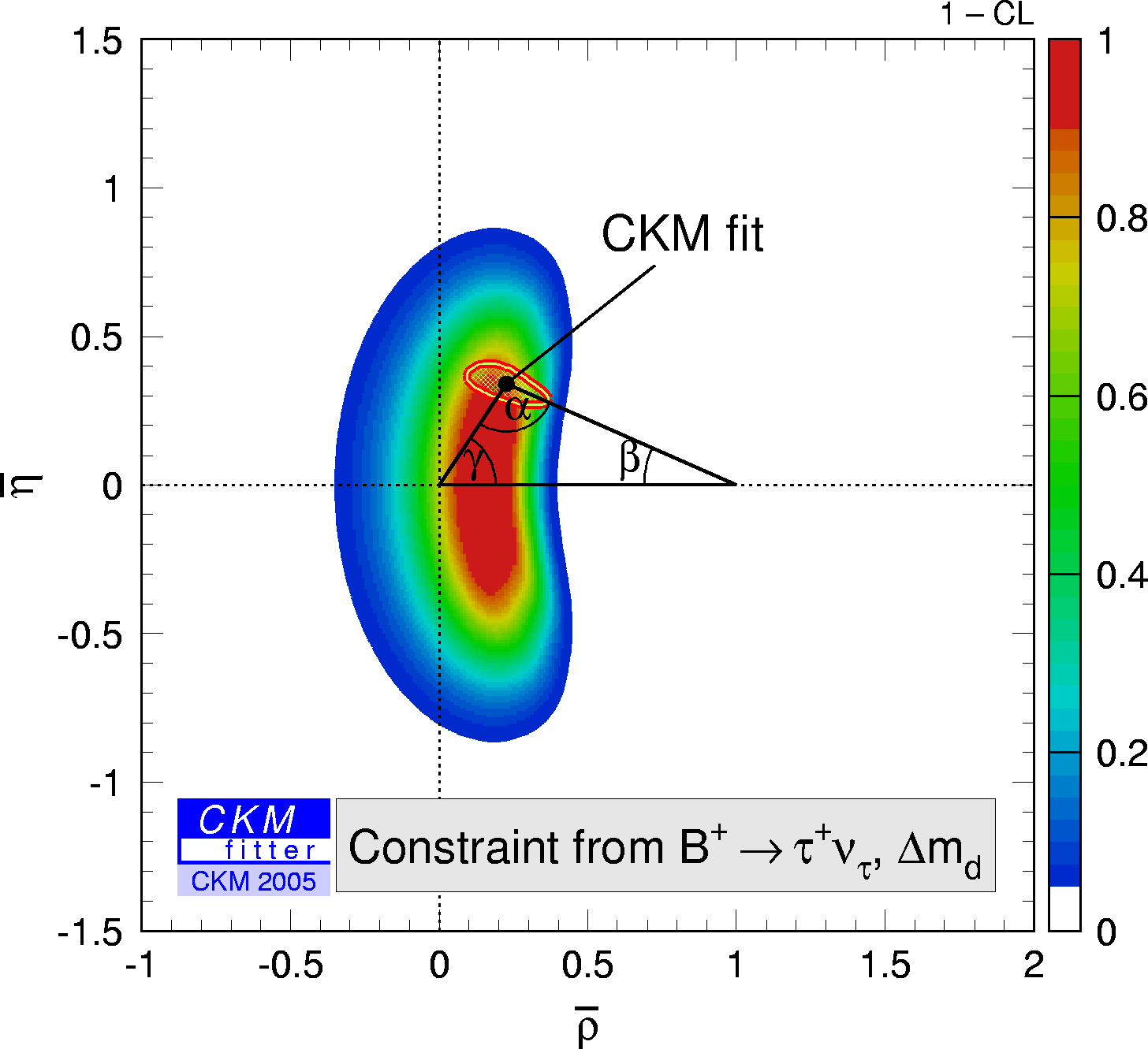eps gif


Constraints on |sin(2β+γ)|

Constrains on |sin(2β+γ)| from the measurement of time-dependent CP asymmetries in D(*) π (ρ). We use the Moriond05 HFAG average as input. The extraction of the UT angles relies on SU(3) symmetry for the estimates of the suppressed-to-leading amplitude ratios. We use
r = 0.019 ± 0.004 and r* = 0.015 + 0.004 / – 0.006 , and apply an additional theoretical uncertainty in form of a 30% error range to these.
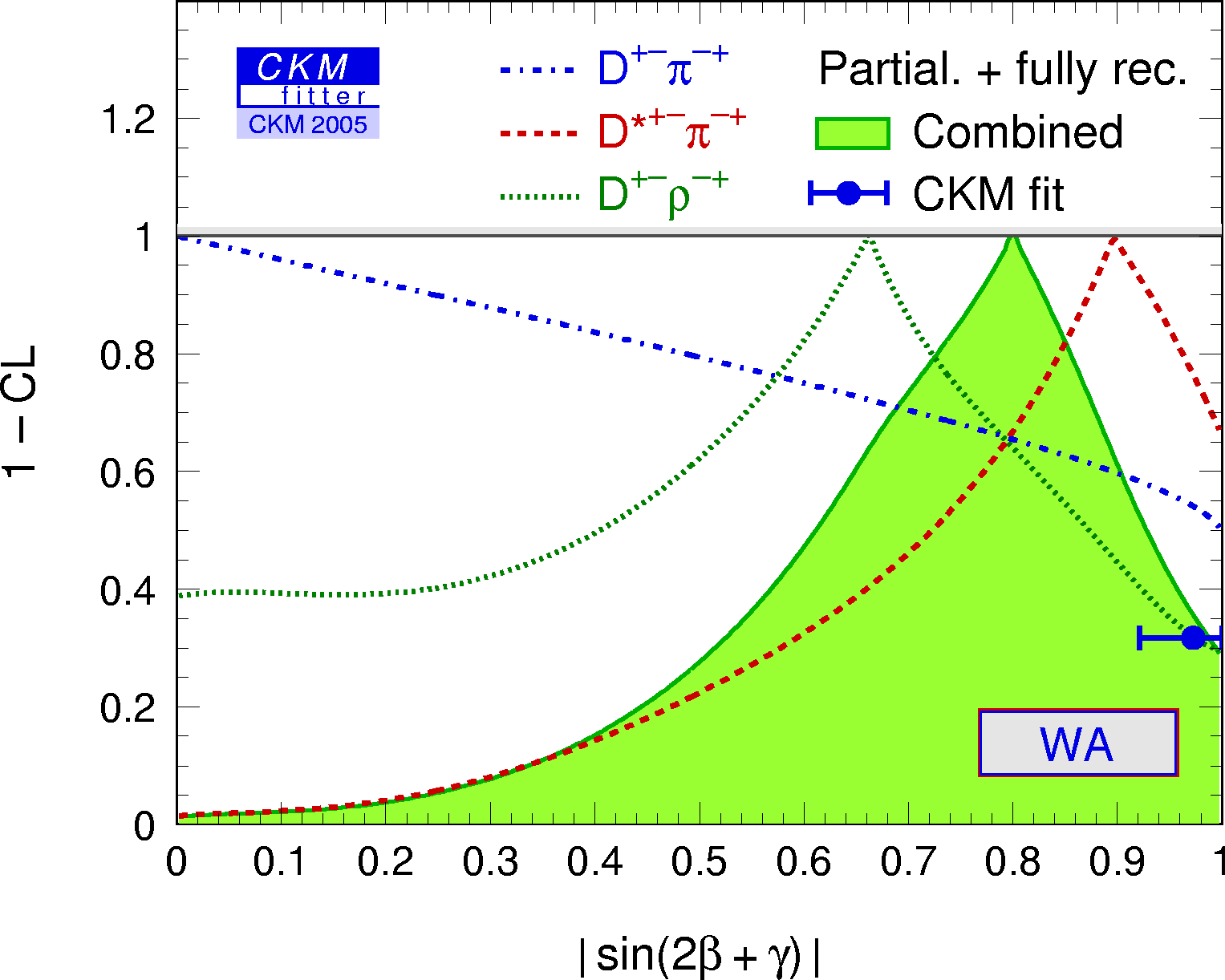eps gif
Translation of this result into γ (using sin(2β) as additional input and choosing among the four solutions to the SM one).
γ[GLW+ADS+GGSZ+|sin(2β+γ)|] = 70 +12 / – 14°
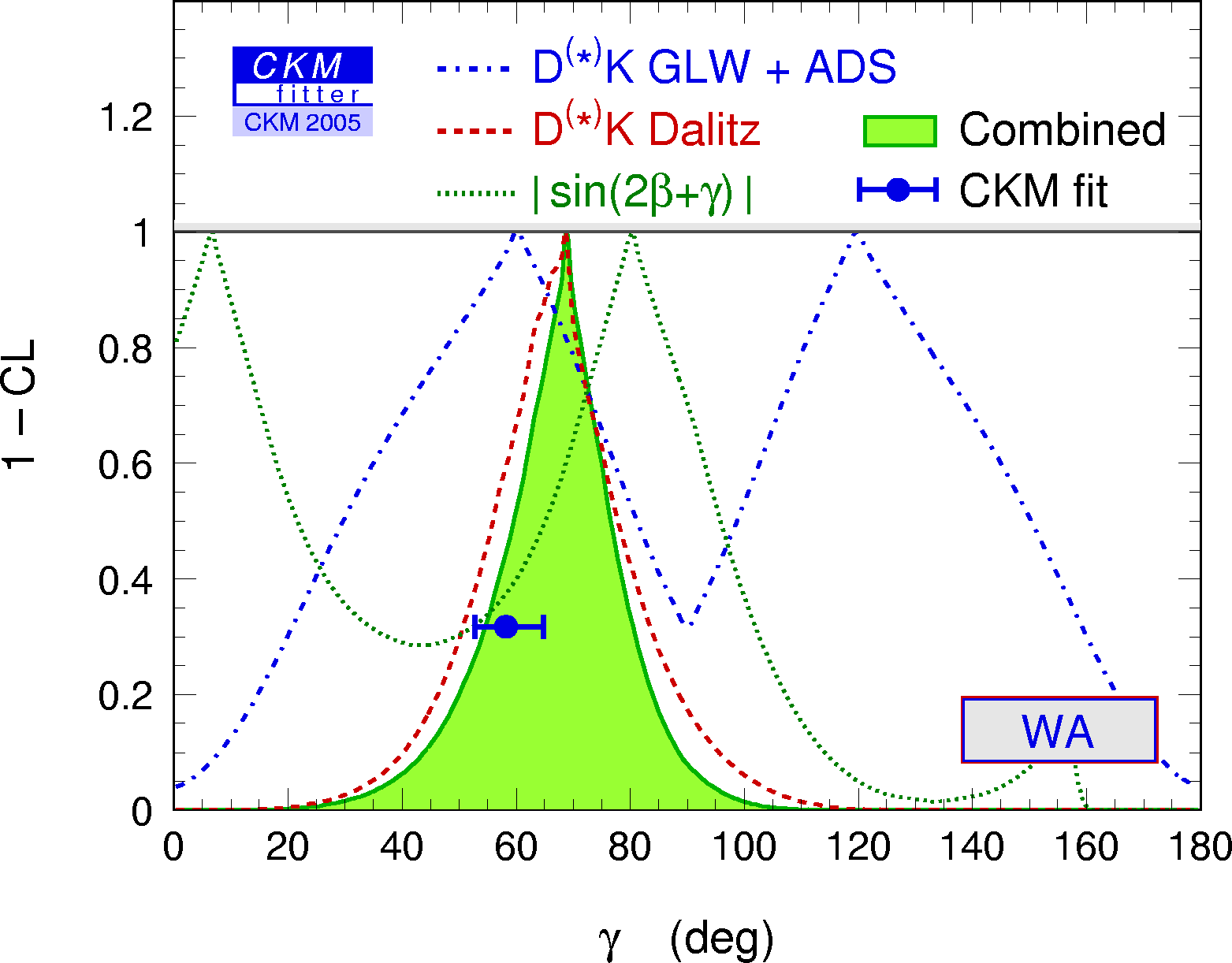eps gif
Constrains from |sin(2β+γ)| in the (ρ-bar,η-bar) plane.
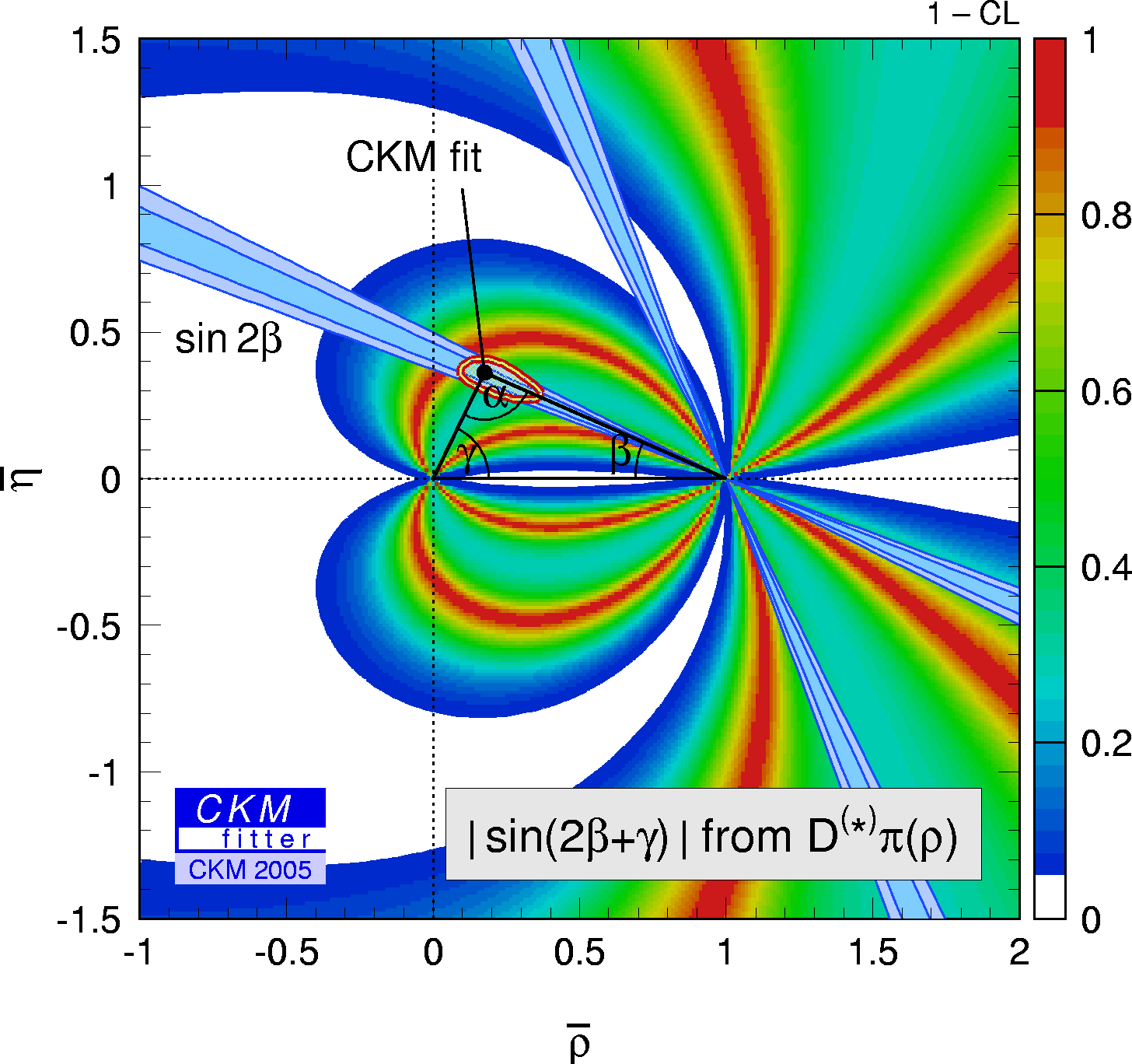eps gif


Contribution of the different input measurement families
to the constraint in the (ρ-bar,η-bar) plane

Constrains from CP conserving quantities (|Vub / Vcb|, Δmd, (Δmd and Δms)) and B+→τ+ ν in the (ρ-bar,η-bar) plane.
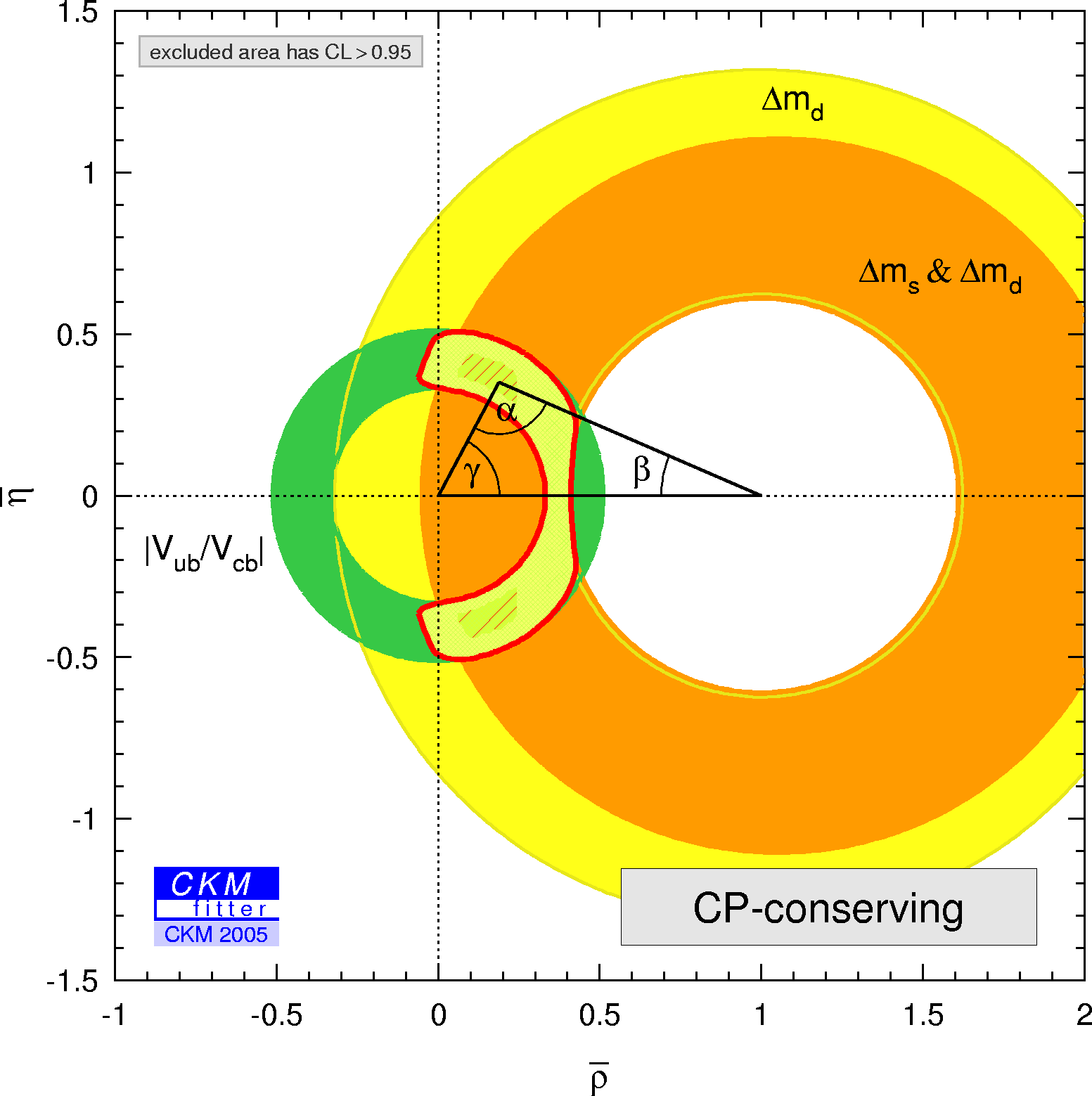eps gif
Constrains from CP violating quantities (sin(2β), α, γ and εk) in the (ρ-bar,η-bar) plane.
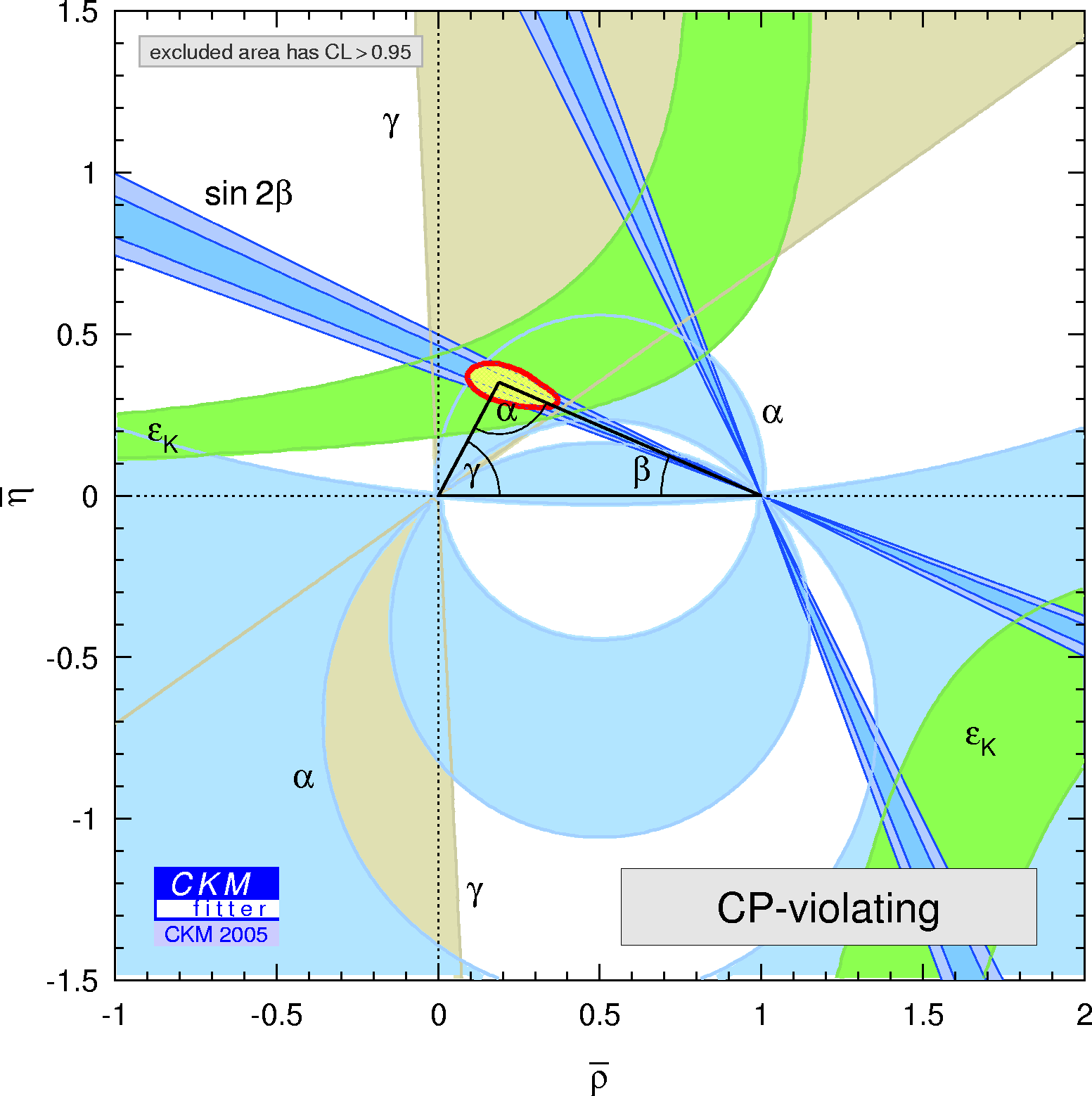eps gif
Constrains from |Vub / Vcb| and s to d transition in the (ρ-bar,η-bar) plane.
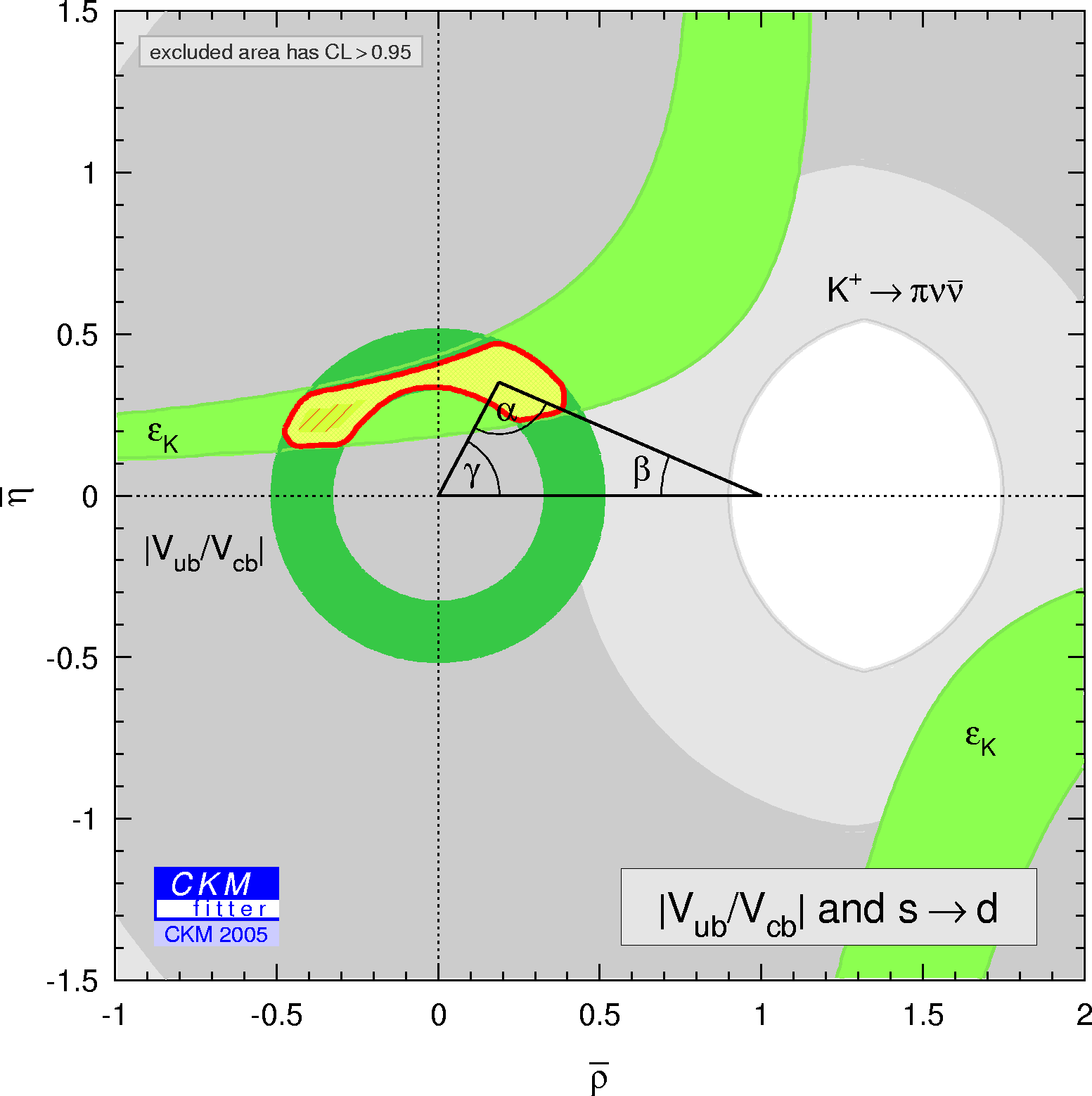eps gif
Constrains from |Vub / Vcb| and b to d transition in the (ρ-bar,η-bar) plane.
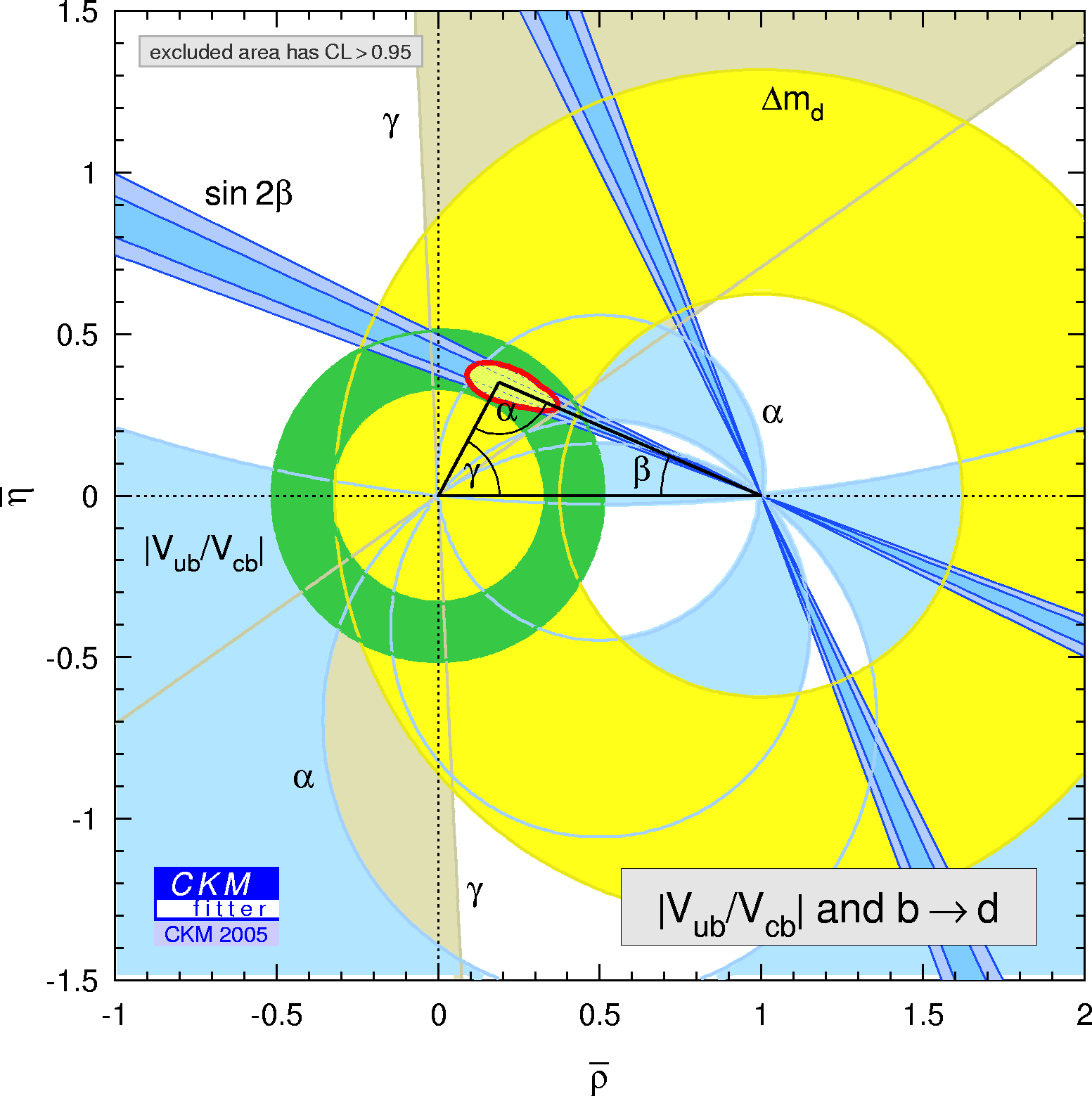eps gif
Constrains from |Vub / Vcb| and b to s transition in the (ρ-bar,η-bar) plane.
eps gif


New physics in B0- B0bar Mixing

New physics in B0- B0bar Mixing can be described model-independently by introducing two new parameters measuring the relative strength (rd2) and the relative phase between the B0- B0bar mixing matrix element containing contributions from SM as well as from NP contributions compared to SM contributions only:
rd2 * exp(i 2*Θd) = <B0 | H[full] | B0-bar>
-------------------------------
<B0 | H[SM] | B0-bar>
Constraints from |Vcb| + |Vub| , Δmd=Δmd{SM} * rd2 and sin(2β+2Θd) in the (ρ-bar,η-bar) plane.
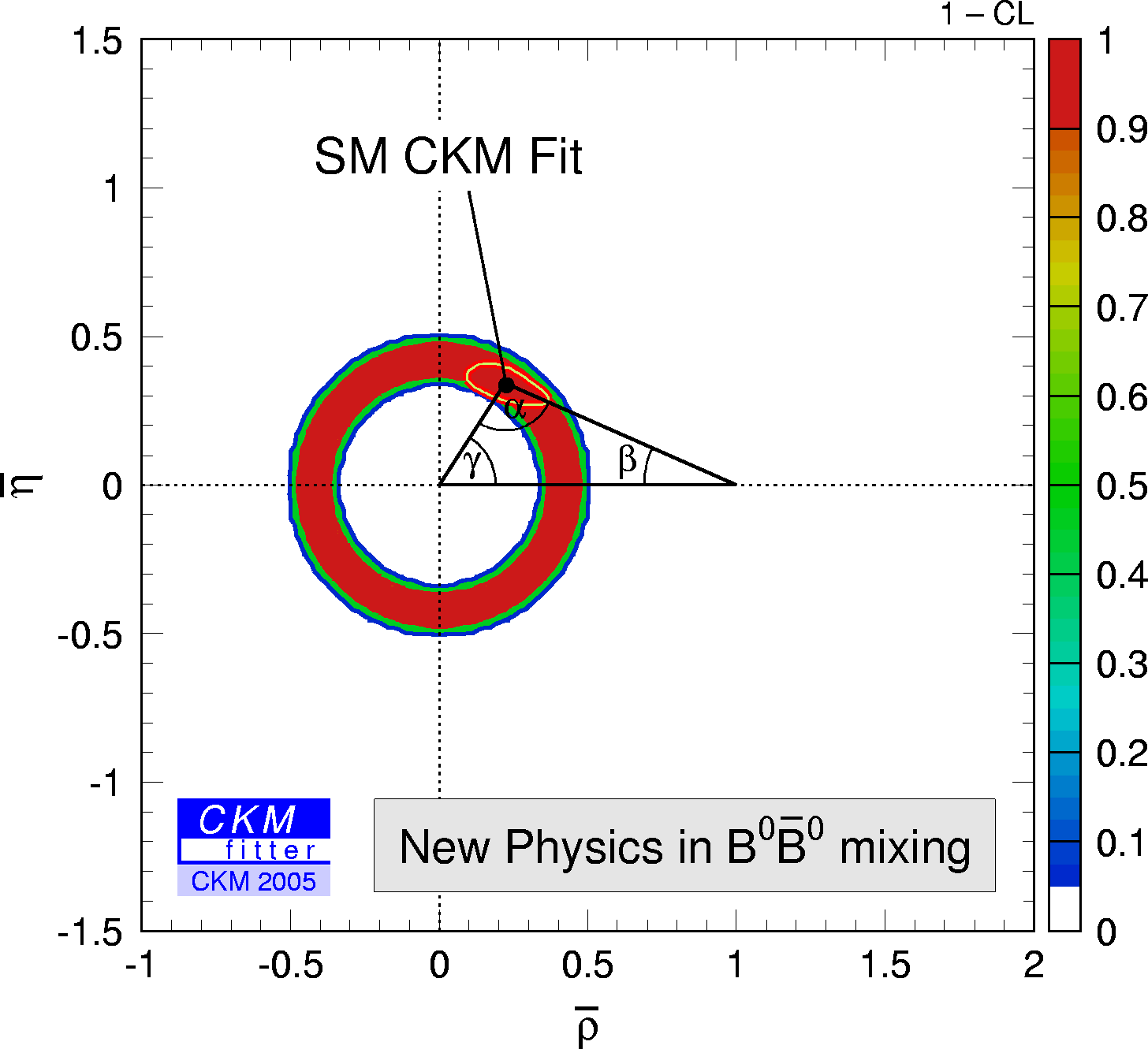eps gif
Constraints from |Vcb| + |Vub| , Δmd=Δmd{SM} * rd2 and sin(2β+2Θd) in the (2Θd , rd2) plane.
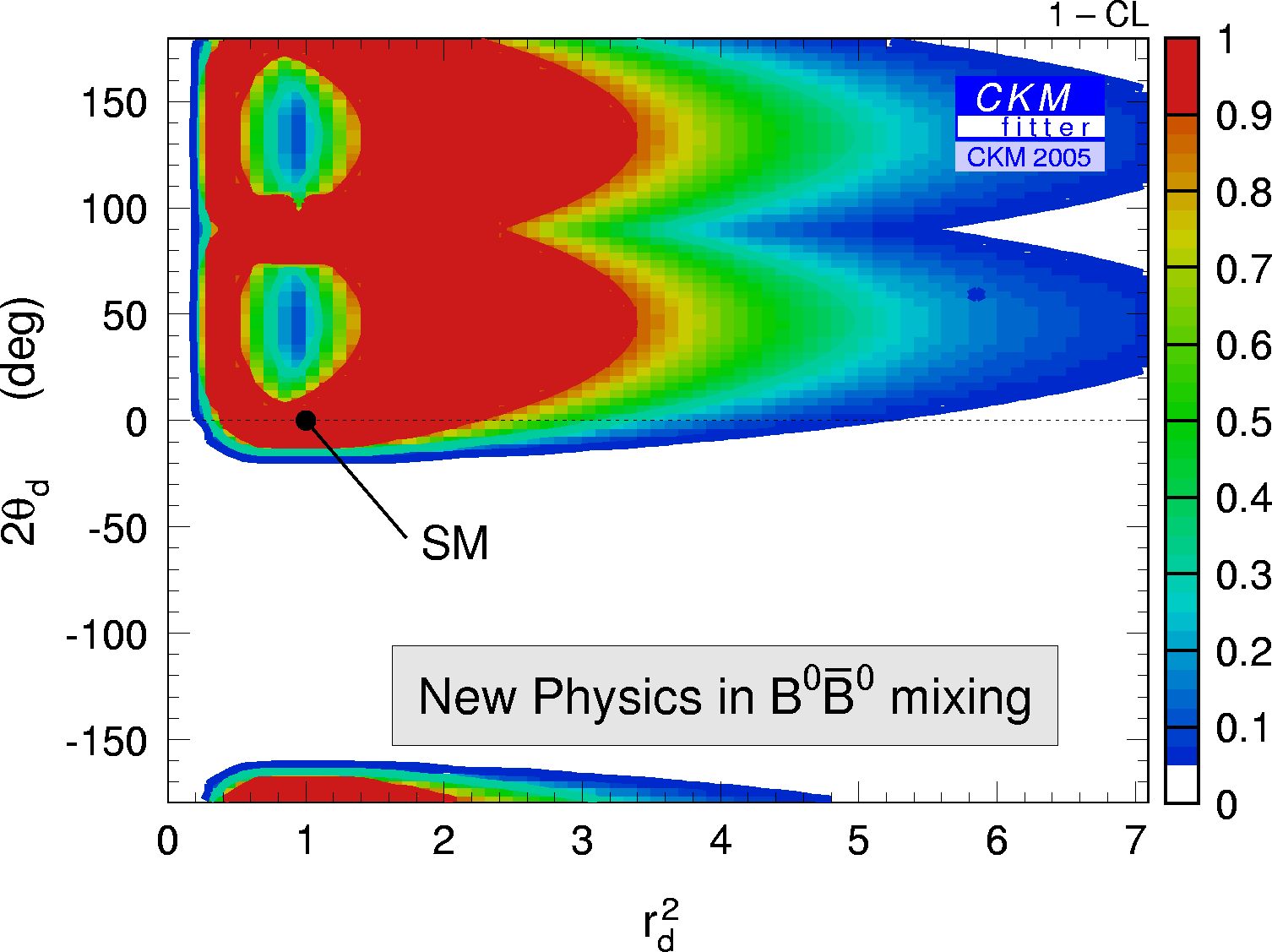eps gif
Since there are four free parameters in the fit (ρ-bar,η-bar,2*Θd,rd2) but only three constraints depend on those there is no other constraint in the (ρ-bar,η-bar) plane visible but the one coming from |Vub| and |Vcb| alone. As a consequence, the allowed region in the (2*Θd,rd2) plane is large.

When using in addition the following inputs:
cos(2β) > 0 (suggested by data), α (ππ, 3π, ρρ), γ the allowed regions are substantially reduced:
in the (ρ-bar,η-bar) plane.
eps gif
in the (2*Θd,rd2) plane.
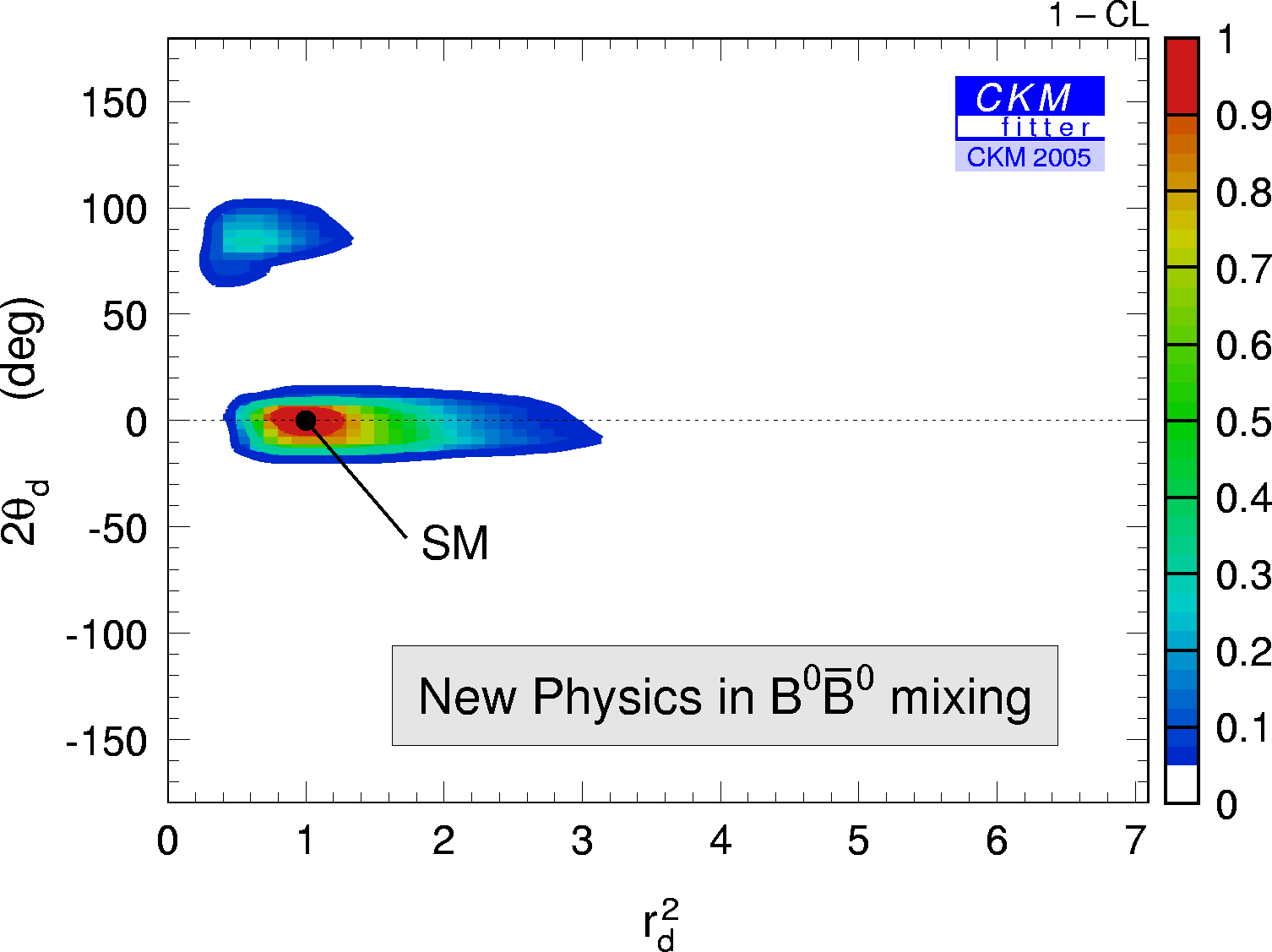eps gif
There are two solutions left. The SM solution (2*Θd = 0 ,rd2 = 1) is prefered. If the other solution can be eliminated with more data the possible additional phase from NP could not be very large (<15 degree). The relative strength of NP to SM contributions can still easily be of order 100%.